In this section you will find several public goods exercises and an illustration how to solve them. The following two examples come from the class notes of Professor Mark Walker from the University of Arizona.

• Costless Public Good: A Water-Level Example
• Costly Public Good: Bug-Spray Examples

We begin with a simple example of a pure public good. Let's suppose there are only two people who live on the shore of Lake Magnavista. Amy likes to water ski and Bev likes to sunbathe. Both activities are seriously affected by the level of the water in the lake. When there is a lot of water in the lake it's good for water skiing, but the water line is so high that there is no beach for sunbathing. When there is much less water, the sunbathing is good but the lake is too shallow for water skiing. Thus, Amy prefers that the lake have lots of water, and Bev prefers that it have much less water. Fortunately, it is possible to raise or lower the water level costlessly, by opening a dam at one end of the lake or at the other end. Unfortunately, it's not clear at what level the water ought to be set.

In order to have a measure of the amount of water in the lake, let's use the water's depth at a specified location on the lake: let x denote the water's depth (in feet) at that location. Amy's and Bev's preferences are described by the following utility functions:

u_A(x, y_A) = y_A - (15 - x)² and u_B(x, y_B) = y_B - 1/2 (6 - x)²,

where x denotes the water level and y_A and y_B are Amy's and Bev's daily consumption of other goods, measured in dollars. Therefore their marginal rates of substitution are:

MRS_A = 30 - 2x and MRS_B = 6 - x.

Amy's most-preferred water level is x'_A = 15 and Bev's is x'_B = 6. Figure 1 depicts their indifference maps. Notice that if the water level is above x'_A, Amy would be willing to pay (i.e., to give up some of the y-good) to have x reduced, and that Bev would similarly be willing to pay to reduce x if it were above her ideal level, x'_B. Suppose Amy and Bev each have incomes of $100 per day.

What makes this situation different than everything we've seen before is that the x-variable can't be at different levels for different people. It's not like tacos or beer, where Amy can have one quantity and Bev another. In this case, the water level can be varied, but it will be the same for both of them. That's why we haven't put A and B subscripts on the x-variable; it's just one variable, not two. The water level in this example is a public good.

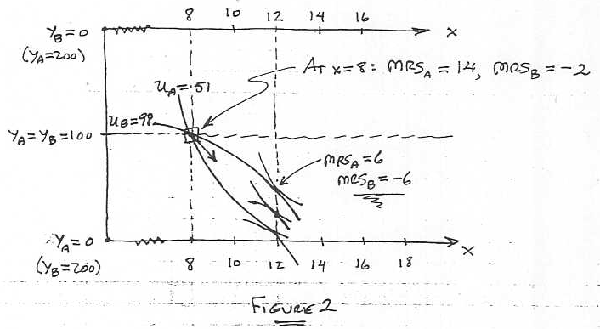
Let's try to determine which outcomes will be Pareto efficient. Figure 2 shows a diagram similar to the Edgeworth Box. The difference is that here in this diagram the "corners" or "origins" for both individuals are placed on the left edge of the diagram, so that when x increases (as we move toward the right), both individuals' consumption of the x-good is increasing. (This is in contrast to the Edgeworth Box, in which, as we move toward the right, Person A's consumption is increasing and B's is decreasing, because with "private goods" like tacos, the more one person gets, the less remains for the other person.)

Let's ask whether a water level of x = 8 feet is efficient. Amy's and Bev's MRS's will be MRS_A = 14 and MRS_B = -2. Amy would pay $14 to increase the water level one foot and Bev would pay $2 to decrease it by one foot -- or Bev would accept $2 as compensation for increasing the water level by one foot. Consequently, if we were to increase the water level by a foot, and if Amy were to compensate Bev by paying her, say, $6, then both would be better off. In fact, you can calculate that Amy's utility will increase from 51 to 58 and that Bev's will increase from 98 to 101 1/2.

So what about the new water level of x = 9 feet? Is it efficient? We have MRS_A = 12 and MRS_B = -3, so we could increase the water level by another foot, with Amy paying another $6 to compensate Bev. The $6 payment is less than the $12 Amy would have been willing to pay, and more than the $3 Bev would have been willing to accept as compensation, so they're again both better off by the one-foot increase with $6 compensation. You can calculate that their utilities will have increased again, to u_A = 63 and u_B = 104.

Now it is becoming clear that as long as Amy would be willing to pay more for an increase than Bev would be willing to accept as compensation, then such an increase, with compensation paid, will make them both better off. In other words, the water level is not efficient so long as MRS_A > -MRS_B --i.e., so long as MRS_A + MRS_B > O. When MRS_A + MRS_B > 0 the marginal social value of an increase in x is positive, so x should be increased. Similarly, we could show that if MRS_A + MRS_B < 0 then x should be decreased (because the marginal social value of an increase is negative).

Thus, the Pareto efficient outcomes are the ones that satisfy the Test Condition MRS_A + MRS_B = O. In our example, it's easy to solve for the efficient water level in the lake: MRS_A + MRS_B = (30 - 2x) + (6 - x) = 36 - 3x; therefore the Test Condition yields 36 - 3x = 0 ; i.e., x = 12. The efficient water level is 12 feet, where MRS_A = 6 and MRS_B = -6.

What if there were more than just two people who use the lake? Each would have some preference involving trade-offs between the desired water level and consumption of other goods, and therefore an indifference map and an MRS schedule, or functon. The Test Condition for Pareto efficiency is the same as with two people: the sum of all the MRS's (i.e., the marginal social value of increasing x) must be zero. If the sum were instead positive or negative, then the water level should be increased or decreased.

Now we know which water level is Pareto efficient. But what level will actually be chosen? That depends on the institutional arrangements by which the water level is chosen. For example, the affected parties might vote on the level they want. The outcome of that institution can be analyzed using game theoretic methods, which we won't do here. Let's suppose instead that Bevowns the lake, or at least that she has the right to choose the water level. What will the water level be? It seems that Bev will choose the level that she likes best, namely x = 6 feet.

But in fact we would expect Bev to bargain or negotiate with Amy to increase the water level (for a price, to be paid by Amy to Bev). Indeed, we would expect them to arrive at a mutually agreeable bargain in which there are no more gains to be had from further trade or bargaining -- i.e., at a Pareto efficient outcome. In our example this means that the water level will be 12 feet, with Amy paying Bev some compensation for the increase from 5 to 12 feet. They will end up at an allocation somewhere in the football-shaped region formed by their initial indifference curves.

What if instead Amy has the right to choose the water level? At first it seems as if she would choose the level she likes best, x = 15 feet. But the same argument as above tells us that again they will negotiate to set the water level at x = 12 feet, but this time with Bev paying Amy some compensation for setting the level below what she would like the most.

It was the economist Ronald Coase who pointed out that the assignment of legal rights will affect only who pays compensation, and how much, but will not affect the level of the externality-producing activity -- in this case, the level of the public good, which is the water level. He was awarded the Nobel Prize for this idea, which has had a profound effect on law and, to a somewhat lesser extent, on legislation.

What if the public good is not costless, as in the water level example? Then the Efficiency Test Condition is that the marginal social value of a one-unit increase in the amount of the public good must be equal to the marginal cost of producing that additional unit -- i.e., that the sum of all the MRS's must be equal to MC:

MRS₁ +MRS₂ + ... + MRS_n = MC

Notice that in our lake example the MC of changing the water level was zero, so this Test Condition is actually the same as the one we used in the example.

Example: Suppose Amy and Bev are plagued by mosquitoes, but it is possible to control the mosquitoes by spraying regularly. The mosquito spray cannot be confined to the property of just one of the women: any spray that is used affects the entire lake shore equally. Suppose Amy's and Bev's preferences are described by the same utility functions as in our water-level example, where x now denotes the number of gallons that are sprayed each week, and y_A and y_B are Amy's and Bev's weekly incomes. If each gallon of spray were free, our problem would be exactly the same as before, because MC = 0. Suppose instead that the spray costs $24 per gallon. Then our Test Condition yields the following:

MRS_A + MRS_B = MC : (30 - 2x) + (6 - x) = 24 i.e., 36 - 3x = 24.

Therefore the Pareto efficient amount of spray is 4 gallons per week, which equates the marginal social value to the marginal social cost. How much will be sprayed if Amy and Bev each choose on the basis of just their own personal benefits from the spray? Bev will not choose to spray at all, because even when x = 0 her MRS is much less than the price of the spray. Amy will choose x = 3 gallons of spray, which equates her own MRS to the price of the spray. Thus, too little spray is chosen. Both women would be better off if an additional gallon were sprayed and, say, Amy paid $22 of the cost and Bev paid the remaining $2.

A More Striking Example: Suppose there are 100 homeowners living on the lake shore, and that each has MRS = 6 -x, where x is the number of tankfuls that are sprayed. Suppose that the cost of the mosquito spray is $100 for every tankful. Then the Pareto efficient amount of spray is x = 5 tankfuls: MRS_i = MC : 100(6 - x) = 100 i.e., 600 - 100x = 100.

How much will actually be sprayed if each homeowner chooses on the basis of just his own benefit from the spray? At x = 0 each homeowner has MRS = 6 -- i.e., each would be willing to pay $6 to increase x to one tankful. But the cost of such an increase -- $100 -- far exceeds each person's MRS. Therefore no one will choose to spray, and even though the marginal social benefit is $600 and the marginal social cost only $100, no spray is forthcoming.