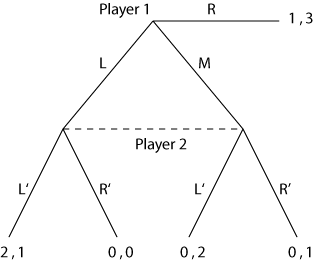
Perfect Bayesian equilibrium (PBE) was invented in order to refine Bayesian Nash equilibrium in a way that is similar to how subgame-perfect Nash equilibrium refines Nash equilibrium. Consider the following game of complete but imperfect information. First, player 1 chooses among three actions: L,M, and R. If player 1 chooses R then the game ends without a move by player 2. If player 1 chooses either L or M then player 2 learns that R was not chosen ( but not which of L or M was chosen) and then chooses between two actions L' and R', after which the game ends. Payoffs are given in the extensive form.

Using the normal form representation of this game given below we see that there are two pure strategy Nash-equilibria - (L,L') and (R,R'). To determine which of these Nash equilibria are subgame perfect, we use the extensive form representation to define the game's subgames. So the game above has no proper subgames and the requirement of subgame perfection is trivially satisfied, and is just the Nash equilibrium of the whole game. So in the game above both (L,L') and (R,R') are subgame perfect Nash equilibria. However, one can see that (R,R') clearly depends on a noncredible threat: if player 2 gets the move, then playing L' dominates playing R', so player 1 should not be induced to play R by 2's threat to play R' given the move.

To strengthen the equilibrium concept to rule out the subgame perfect Nash equilibrium (R,R') we impose the following requirements.

R1: At each information set, the player with the move must have a belief about which node in the information set has been reached by the play of the game. For a nonsingleton information set, a belief is a probability distribution over the nodes in the information set; for a singleton information set, the player's belief puts one on the decision node.

R2: Given the beliefs, the players' strategies must be sequentially rational. That is at each information set the action taken by the player with the move (and the player's subsequent strategy) must be optimal given the player's belief at the information set and the other players' subsequent strategies ( where a "subsequent strategy" is a complete plan of action covering every contingency that might arise after the given information set has been reached).

R3: At information sets on the equilibrium path, beliefs are determined by Bayes' rule and the players' equilibrium strategies.

R4: At information sets off the equilibrium path, beliefs are determined by Bayes' rule and the players' equilibrium strategies where possible.

In our example R1 implies that if the play of the game reaches player 2's non-singleton information set then player 2 must have a belief about which node has been reached (or equivalently, about whether player 1 has played L or M). This belief is represented by probabilities p and 1-p attached to the relevant nodes in the tree. Given player 2's belief, the expected payoff from playing R' is p x 0 + (1-p) x 1 = 1-p . The expected payoff from playing L' is p x 1 + (1-p) x 2 = 2 - p. Since 2 - p > 1-p for any value of p, requirements 2 prevents player 2 from choosing R'. Thus, simply requiring that each player have a belief and act optimally given this belief suffices to eliminate the implausible equilibrium (R,R').

Requirements 1 and 2 insist that the players have beliefs and act optimally given these beliefs, but not that these beliefs be reasonable. Requirement 3 imposes that in the subgame-perfect Nash equilibrium (L, L') player 2's belief must be p=1; given player 1's equilibrium strategy (namely, L), player 2 knows which node in the information set has been reached. As a second hypothetical illustration of Requirement 3, suppose that in the game above there was a mixed strategy equilibrium in which player 1 plays L with probability q1, M with probability q2, and R with probability 1-q1-q2. Then requirement 3 would force player 2's belief to be p = q1/(q1+q2).

Requirements 1 through 3 capture the essence of a perfect Bayesian equilibrium. The crucial new feature of this equilibrium concept is due to Kreps and Wilson (1982): beliefs are elevated to the level of importance of strategies in the definition of equilibrium. Formally an equilibrium no longer consists of just a strategy for each player but now also includes a belief for each player at each information set at which the player has the move. Finally, a perfect Bayesian equilibrium consists of strategies and beliefs satisfying requirements 1 through 4.